Desargues Theorem in a Finite Geometry

Prepared by:

Joseph Malkevitch
Department of Mathematics and Computer Studies
York College (CUNY)
Jamaica, New York

email:

malkevitch@york.cuny.edu

web page:

http://york.cuny.edu/~malk/

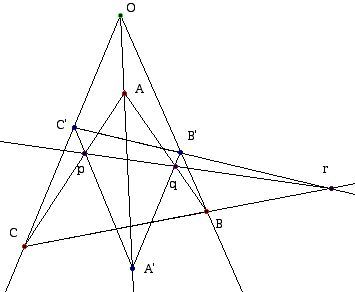
Desargues "theorem" is one of the most unexpected and elegant results in projective geometry.

Given two triangles ABC and A'B'C', if AA', BB', and CC' are lines which go through a single point O, then the points where AB and A'B', BC and B'C', and AC and A'C' meet lie on a line (are collinear)!
If the roles of point and line above are interchanged, than we get the dual of Desargues "statement" which amazingly is also the converse of the original statement. Desargues statement is true for any projective plane embedded in a projective 3-space, for the real projective plane, and for finite projective planes which arise by using coordinates from a field (in particular from a Galois Field). However, there are projective planes which fail to obey the Desargues statement. Such planes can occur for either infinite planes or for finite planes. One nice example of a non-desarguesian plane (in the infinite case) is the Moulton Plane:

http://en.wikipedia.org/wiki/Moulton_plane

The basic idea here is that the lines of the Euclidean plane are modified so that if they have positive slope they are "bent" when they cross the x-axis. (It is a bit like what happens to light rays when they enter water from the air - they bend. However, in the Moulton Plane only the lines of positive slope are "bent.")

Here is an example (exercise) which may help enrich your understanding of the real projective plane, Euclidean analytical geometry, and the projective geometry of finite planes. If you work out the details it will help you see how Desargues Theorem holds in finite geometries where the coordinates come from a finite arithmetic (finite field; Galois field).

We will work in Z₅ which will denote the integers mod 5, and which form a field. We can think of the elements of this field as the "numbers" 0, 1, 2, 3, and 4. (We will drop the convention of using bars over these numbers or showing them in a different type font to distinguish them from "ordinary" integers.)

Hence, 4 + 3 = 2 since 7 leaves a remainder of 2 when divided by 5 and 4(3) = 2 since 12 divided by 5 leaves a remainder of 2. "1/2" = 3 since 2x3 is congruent to 1 mod 5. (Thus, we can solve the equation 2x=1; x =3 solves it.)

Our points are the ordered triples (x, y, z) where not all the coordinates are zero and (kx, ky, kz) is the same point as (x, y, z) when k is not 0. The only legal values for x, y, and z are 0, 1, 2, 3, or 4. Similarly we use as lines ax +by +cz = 0. (Again a, b, c are not all 0 and there are only 5 choices for each of them: 0, 1, 2, 3, or 4.)

Suppose we are given the line x + y + 2z = 0. There are only a finite number of points (exactly 6 , in fact) on this line. Think of the corresponding "affine" (Euclidean) line: x + y = 3, (y = 4x + 3) obtained by setting z = 1. Since -2 is congruent to 3 mod 5, we get the above equation. So we get 5 points by letting x be 0, 1, 2, 3, and 4 in turn. There is also one more point on this line: the point where the line meets the line at infinity (z=0) which is gotten by setting z = 0 in the original 3-variable equation.

(0,3, 1)
(1,2, 1)
(2,1,1)
(3,0,1)
(4,4,1)

and the point at infinity on this line (last coordinate 0)

(1,4,0). (This "makes sense" because the line has slope 4.)

What is exciting about these finite planes is that one can write down all the points, all the lines, all the points on each line, and all the lines through each point if one has the patience. To test your skill in this 31 point world, you can try verifying Desargues Theorem for this pair of triangles:

A = (1,2,1)

B = (2,1,1)

C= (3,2,1)

and

A' = (2,4,1)

B' = (1,3,1)

C' = (1,4,1)

First, check that AA', BB', CC' go through a single point. Second, check that AB intersect A'B', AC intersect A'C' and AC intersect A'C' at points that all lie on a single line!

To do this you may want to use the "determinant" approach to finding the line through two points and the point where two lines meet. Mathematically, one does the same thing because we are working in a projective geometry and lines can not be parallel. Lines can be thought of as having equations or coordinates, and similarly for points.