Points, Lines, and Duality

Prepared by:

Joseph Malkevitch
Department of Mathematics and Computer Studies
York College (CUNY)
Jamaica, New York 11451

email:

malkevitch@york.cuny.edu

web page:

http://york.cuny.edu/~malk/




Introduction

Points and lines are the building blocks of shapes of all kinds. As a result, they are of interest to those concerned with geometry as a branch of physics and as a branch of mathematics. If one picks 3 points in physical space one can ask the question: Do the angles determined by the lines which connect these three points at the vertices of the triangle formed by the points add up to 180 degrees? This is a question about physics. On the other hand, reasoning deductively from a modern collection of axioms (say those due to David Hilbert)) that form a basis for the branch of mathematics concerned with Euclidean geometry makes can conclude with certainty that the sum of the angles in at the vertices of a triangle add to 180 degrees.

Two fundamental facts about Euclidean geometry are:

1. Two points lie on one and only one line.

2. Two lines intersect in at most one point.

The reason for 2. is that lines can either intersect or be parallel in the Euclidean plane. A modern version of Euclid's famous Fifth Postulate (axiom) is that given a point not on a line l there is exactly one parallel to l which contains the point P.

Is the geometry of the physical world Eucldean? As a question in Pbysics the aswer is that we do not know. The work of J. Bolyai, N. Lobachevsky, and G. Riemann showed that there are consistent geometries in which there are unique parallels though a point to a given line, no parallel to a line through a given point, and many parallels.

One route to the geometry where there are no parallels was found by the artist-mathematicians of the Renaissance who noted that when lines which seem to be parallel in physical space appear to get closer when the lines are viewed in the distance. We are all familiar with the illusion that railroad tracks look as if they meet in the distance but in fact remain parallel. These artists employed a technique called perspective drawing which made scenes look more realistic by having lines which are parallel appear to meet on a special line called the vanishing line or horizon line. These ideas have the seeds of what today would be called projective geometry.

Two fundamental facts about projective geometry are:

1. Two points lie on one and only one line.

2. Two lines intersect in exactly one point.

Note that Euclidean and projective geometry agree on the first statement, and that the second statement is a rewording of the notion that there are no parallel lines.

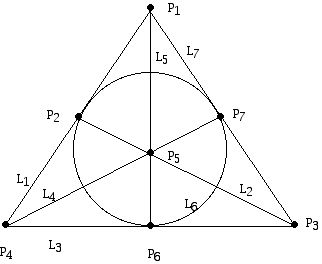
The diagram below illustrates a very simple example of a projective geometry, albeit one which seems strange to many people because it has lines with only a finite number of points on a line, and the total number of points in the geometry is finite.

The geometry above is known as the Fano Plane, and it is named for Gino Fano, an Italian geometer who was a pioneer in the study of finite geometries.
Note that there are 7 points and 7 lines in this geometry. Since there are 7 points there are 21 pairs of points and you can check that there is always exactly one line which goes through this pair. For example, if one chooses the pair P₆ and P₇ the line which goes through them is L₆. You can also check that each pair of lines meet in exactly one point. The lines above all lie along lines that exist in the Euclidean plane with one exception. This is the line labeled L₆. This line looks like a circle. It can be proved that no matter how one tries to represent the Fano plane in the Euclidean plane that one can not represent all 7 of the liness in the Fano plane so that they lie along straight line. The best one can do is something like the diagram above, where one "line" does not lie along a Euclidean line.