Geometric Structures

Prepared by:

Joseph Malkevitch
Department of Mathematics and Computer Studies
York College (CUNY)
Jamaica, New York 11451

email:

malkevitch@york.cuny.edu

web page:

http://york.cuny.edu/~malk/


Session 2

Euclidean geometry, which evolved from Euclid's seminal work, is a very complex mathematical system. In its modern form Euclidean geometry is spelled out by the system which David Hilbert worked out in the early 20th century. From the standpoint of intellectual history, a critical role was played by Euclid's 5th Postulate. This axiom, which as Euclid stated it was more complex than the others Euclid gave, for centuries created speculation that it was derivable as a theorem from the other axioms he stated stated. However, by the late 19th century, due to the work of Bolyai, Gauss, Lobachevsky, and Riemann, it was clear that Euclid's 5th postulate is independent of his other axioms, that is, there are "geometrical games" where there are unique parallels, no parallels, and many parallels through a point to a given line. Thus, the statement that the sum of the angle measures of a triangle is 180 degrees is a theorem in Euclidean geometry, but not in Bolyai-Lobachevsky geometry. Many of the properties we take for granted about the Euclidean plane fail to hold in the classical Non-Euclidean geometries.

The distinctions between Euclidean geometry and the classical Non-Euclidean geometries can be discussed in a very simplified way using the following axioms.

1. If P and Q are two points there is a unique line which contains P and Q.

(One can think of points as being a set and lines as being sets of points or one can also use language where points are a set and there is an incidence relation which gives information about which points are "on" which lines.)

2. If l and m are lines there is at most one point where l and m intersect.

3. If P is a point not on a line l, there exists a unique line m which is parallel to m and passes through P.

4. There exist 4 points, no three collinear.

(Points are collinear if they lie on the same line. Lines are concurrent if they pass through a single point.)

A model for an axiom system is given by creating an "interpretation" for the undefined terms in the system. In a model one specifies what the points are and what the lines are. For example, one can say points are pairs (x, y) where x and y are real numbers and lines are equations of the form Ax + By + C = 0 where A,B, and C are real numbers and A and B are not both 0.

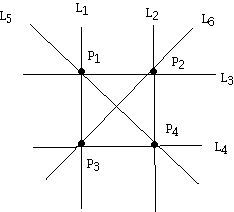
A geometry which obeys these 4 axioms but has only only 4 points and 6 lines can be defined based on the diagram below. Note that lines L₅ and L₆ have no point in common and are, thus, parallel.

The geometry defined by the figure above has 3 classes of lines where the lines within a class are parallel but those from different classes intersect. In Euclidean geometry the parallel classes correspond to the lines with different slopes (and with undefined slope).

If we replace Axiom 3 by:

3'. Given two lines l and m there is a unique point P which is contained in both lines

we can verify that the diagram below satisfies these axioms:

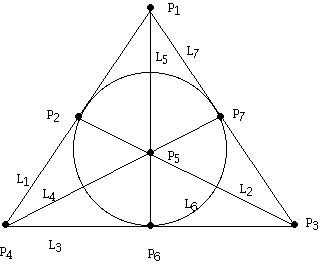
This geometry which has 7 points and 7 lines (each of which consists of 3 points) is known as the Fano Ppane. It is named for Gino Fano, who was a pioneer in the area of finite geometries. Notice that there is one line that in the diagram looks like a circle rather than having points that lie along some Euclidean line. (It can be shown that there is no way of representing the Fano plane in the Euclidean plane so that the points lie on 7 "straight" lines.")

If we replace Axiom 3 by Axiom 3'':

If P is not on line l there are many lines through P parallel to l.

The diagram below consisting of 5 points and 10 lines is a model for our 4 axioms. It is not difficult to verify that through any point P not on line l there are precisely 2 parallels to l though P. (Remember that in the diagrams above and below the only points are the dark dots or unfilled in small circles.)

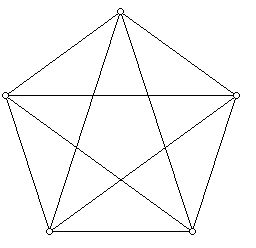
It turns out that finite geometries such as those defined by the three diagrams above are a fascinating class of objects. We will see that there is a nice connection between ideas in Modern Algebra (finite or Galois fields) and finite geometries.