Practice for the Final Examination
Prepared by:
Joseph Malkevitch
Mathematics Department
York College (CUNY)
Jamaica, NY 11451
email:
malkevitch@york.cuny.edu
web page:
http://york.cuny.edu
1. Given the election below:
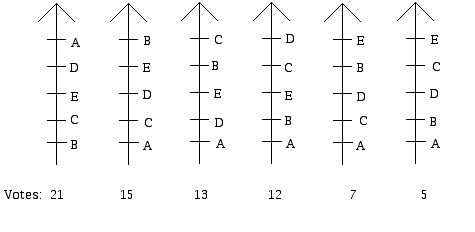
i. Compute the pairwise vote matrix for the election above.
ii. Decide the winner (if there is one) of the election using:
a. Plurality
b. Run-off
c. Sequential Run-off
d. Borda Count
e. Condorcet
f. Sequential run-off based on the Borda Count (Baldwin)
g. Coombs
iii. Be prepared to give a brief account of Arrow's Theorem and why it is important.
2. i. Use Adams, Jefferson, and Webster to apportion a legislature of 15 seats. Each of three regions is entitled to a minimum of at least one seat. The populations of these regions is shown below. The apportionment should be done "twice:"
a. Using a divisor and rounding (state what divisor you used so that when you rounded as the method requires that 15 seats were distributed). (If a tie occurs when 15 seats are given out, say so.)
b. Using the table method check if you get the same answer as you did for above for Adams, Jefferson, and Webster. For each of these methods indicate the order in which the seats are given out via the table method; when there is a tie, indicate this by having the states share these seats. Thus, here for example, seats 1-3 will be shared.
|
|
A
|
B
|
C
|
|
Population
|
12300
|
9600
|
8100
|
ii. Use Hamilton's method to apportion 15 seats using the data above.
2. Given the weighted voting game: [14; 10, 7, 6, 3]
a. List the minimal winning coalitions
b. Determine the Shapley power of each of the players.
c. Determine the Banzhaf power of each of the players.
d. Suppose that secretly the players with weights 6 and 3 agree to always vote together. What are the power relations now as indicated by the Shapley and Banzhaf power indices? (Hint: What is the 3 player game that is now really being played?)
4. Consider the zero-sum games, with payoffs shown from Row's point of view below:
a.
|
|
Column I
|
II
|
III
|
|
Row 1
|
3
|
-1
|
4
|
|
2
|
-2
|
0
|
5
|
b.
|
|
Column I
|
II
|
III
|
|
Row 1
|
2
|
-3
|
3
|
|
2
|
-4
|
5
|
-1
|
i. What is the best-worst strategy for Row and Column in each game?
ii. Does either game have dominating strategies?
iii. Does either game have a saddle point?
iv. Find the optimal way to play these games for each player. (This means considering issues of dominating strategies or finding a saddle point to find a value for the game, and/or find a small matrix for which one can design optimal spinners.) As part of your solution what is the payoff to each player.
5. Find optimal spinners and the value of the game for the following zero-sum game:
Payoffs below are from Row's point of view.
|
|
Column I
|
Column II
|
|
Row 1
|
7
|
-4
|
|
Row 2
|
-2
|
1
|
6. Find the pure and/or mixed Nash equilibrium (equilibria) for the non-zero-sum game below:
|
|
Column I
|
Column II
|
|
Row 1
|
(7,7)
|
(-1, 6)
|
|
Row 2
|
(6, -1)
|
(0, 0)
|
Is this a familiar game?
7. For the bankruptcy situations below, find what amount from E is given to each player using:
a. Equality of gain
b. Equality of loss
c. Maimonides gain
d. Maimonides loss
e. Shapley value
f. Proportionality
g. Contested garment rule ("Talmudic method") (Only use this when there are two claimants)
i. E = 240; A claims 80; B claims 200
ii. E = 240; A claims 180; B claims 220
iii. E = 240; A claims 40; B claims 300
iv. E = 240; A claims 60; B claims 140; C claims 200
