Notes on Modeling: Session III: Voting Methods and Arrow's Theorem

Prepared by:

Joseph Malkevitch
Mathematics Department
York College (CUNY)
Jamaica, NY 11451

email: 

malkevitch@york.cuny.edu

web page: 

http://york.cuny.edu/~malk/

1. We are now getting to see what a complex environment voting and elections is. There are many situations where decisions are made and they take place in a large range of voting environments (committee meetings, UN Security Council, EU decisions, US Senate, votes for Mayor). Sometimes there is only one position to be filled and sometimes many. Sometimes one desires the alternatives to be ranked (with or without ties) and sometimes one wants to choose a single winner.

2. If we restrict ourselves to the case of electing a single winner, there are many appealing methods provided we move from the standard ballot to an ordinal ballot. However, when there are three or more candidates we see over and over again that different reasonable sounding methods (plurality, run-off, sequential run off, Borda, Condorcet) can yield different winners. On what basis should be say that one method is superior to another?

3. It was this issue that Kenneth Arrow, a mathematically trained economist, attempted to resolve by listing a collection of fairness rules that he thought would be desirable for any election decision method which started with ordinal rankings (ties allowed) and selected a ranking for society (ties allowed). He sought a firm footing for how individual preferences could be resolved into a choice for society. Arrow won the Nobel Memorial Prize in Economics for his extraordinary work in this area. His famous theorem was part of doctoral dissertation at Columbia University.

Arrow's result was that there was no method using ordinal ballots (three or more alternatives to choose from) that obeyed a short list of fairness rules. Arrow's work set off an explosion of interest in what has come to be known as "social choice theory." Among the more important developments were:

a. Will other types of ballots (yes-no; approval ballot, range ballot) allow one to "get around" the implication of Arrow's Theorem?

b. Are there election decision methods that encourage voters to be sincere in the way they fill out there ballots, or can all voting systems be "manipulated?"

c. What are the consequences of having available polling data about voter preferences for electoral candidates? Does such data encourage voters in group X to alter their sincere ballots, and use their knowledge of what decision method is to be used to improve the outcome from X's point of view?

d. Are there other fairness axioms other than those mentioned by Arrow which would enable decision makers to see the pros and cons of using particular election decision methods?

4. Among the ideas that Arrow considered and that have been greatly extended subsequently are:

a. Monotonicity (more support should not cause harm) There are many ways one can formalize what "more support" means. Similarly less support should not mean a candidate does better.

b. Independence of Irrelevant Alternatives

c. If more voters with a preference schedule whose top choice is alternative A arrive to vote this should not harm A.

d. Clones Suppose there is a liberal candidate and a conservative candidate seeking office, where the conservatives are aware that the liberal has more support. The conservatives, knowing that plurality voting will be used, might try to get another liberal to run for office so as to split the liberal vote. James Buckley (brother of William Buckley) became Senator from NY in an election where two well known and popular "liberal" candidates split the liberal vote. This allowed the much more conservative Buckley, who was unknown to a lot of the voters to gain office.

5. Often discussions of "strategic voting," the idea of voting in a way that uses information one might have about voter preferences in addition to the decision method being used, exploits examples that show unintuitive behavior associated with particular fairness axioms to design a voting strategy.


6. Example: For the Borda Count, if one knows that there are three candidates and A and B are the most popular in a close race, you may feel that you can help your first choice, A, by voting for C in second place, rather than B in second place, even though your honest ballot would be:

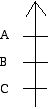
Since C has little chance of getting elected and by using the Borda Count, putting B in second place helps B, strategic voting might help your candidate A if you use the ballot: